本研究の一部は, JKA RING!RING プロジェクト 平成23年度機械工業振興補助事業の支援によるものである.
■Reconstruction Method of Trimmed Surfaces Maintaining G1-Continuity with Adjacent Surfaces
Yuta MURAKI, Katsutsugu MATSUYAMA, Kouichi KONNO and Yoshimasa TOKUYAMA
ABSTRACT
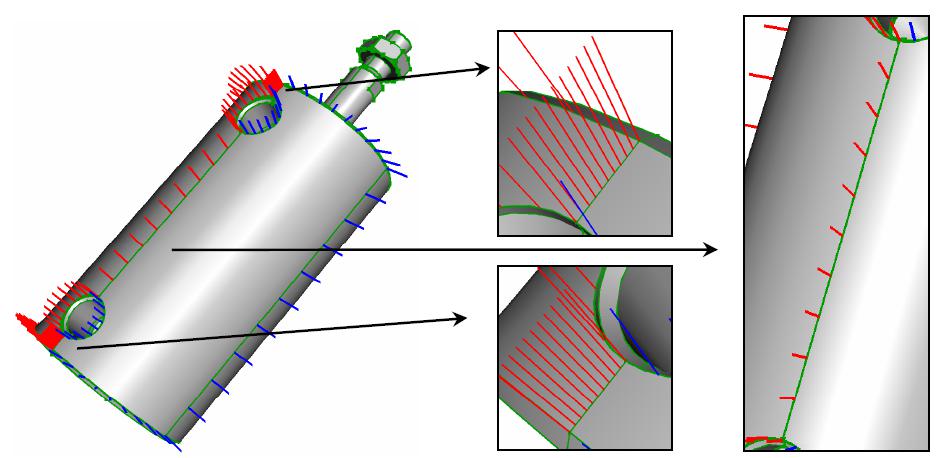
In 3D CAD systems, reconstruction of trimmed surfaces is one of the important research topics. When 3D CAD data is exchanged between different CAD systems, it does not deliver the specifications of CAD systems completely. Therefore, we have to correct the CAD data, such as moving the point in order to fill gaps. Generally, CAD data is expressed by set of trimmed surfaces. Then, we need to modify the trimmed surfaces. It is necessary, however, to modify the trimmed surface shape so as to maintain geometrical consistency of the boundary edges and surfaces in direct modeling, and this is a big restriction. It is effective to apply a new free-form surface to a closed region enclosed with the modified edges because the consistency of a trimmed surface can be maintained. Many shapes with holes or concave shapes are included in CAD data. Moreover, it is necessary to consider maintaining
G1-continuity with adjacent surfaces, but it is difficult to reconstruct the trimmed surface by the conventional surface fitting method.
In this paper, we propose the reconstruction method of trimmed surfaces with maintaining G1-continuity with adjacent surfaces. In the proposed method, boundary edges are input and surfaces are output. Two surfaces are connected with G1-continuity by using the control points at the connection section obtained from cross boundary derivatives. In addition, the control points in the region or on the discontinuous boundaries are obtained by approximating sample points, and the proposed method can also be applied to shapes with holes or concave shapes.

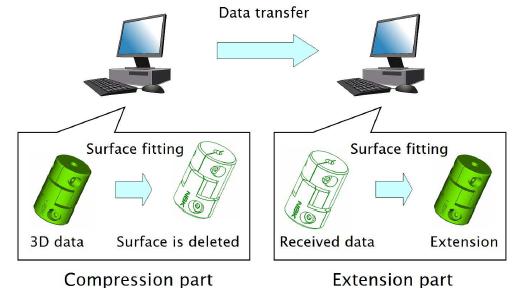
■Evaluation of 3D Data Compression and Retrieval
Method Based on Curve Mesh Filling
Gulibaha Silayi, Tsutomu Kinoshita, Yuta Muraki, Katsutsugu Matsuyama, Kouichi Konno
Digital mock-up (DMU) and/or compound document with 3D data are one of the most important methods to represent a product model. Since the data size of 3D models becomes greater year by year, 3D data compression and retrieval algorithm is required to easily exchange such information through the Internet. Because broadband is still not popular in some regions in the world, it is necessary to send huge data in a small size as much as possible. Therefore, in this paper we developed an application system that transfers 3D surface models and then the performance of the 3D surface compression and retrieval algorithm is evaluated.
